| | An arc of a circle is a "portion" of the circumference of the circumvolve. The length of an arc is merely the length of its "portion" of the circumference. The circumference itself can be considered a total circumvolve arc length. |  | |  | Arc Measure: In a circle, the degree measure of an arc is equal to the measure of the fundamental angle that intercepts the arc. | |  | Arc Length: In a circle, the length of an arc is a portion of the circumference. The letter "south" is used to represent arc length. | | 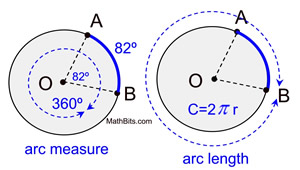 | Consider the following proportion: 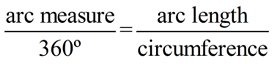 | If we solve the proportion for arc length, and replace "arc measure out"
with its equivalent "cardinal angle", we can plant the formula: 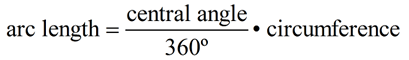 Notice that arc length is a fractional part of the circumference. For example, an arc measure of 60º is one-sixth of the circle (360º), so the length of that arc will be one-sixth of the circumference of the circle. 
In circle O, the radius is 8 inches and small-scale arc 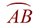 is intercepted by a key angle of 110 degrees. Observe the length of minor arc is intercepted by a key angle of 110 degrees. Observe the length of minor arc 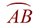 to the nearest integer. to the nearest integer.

As you progress in your study of mathematics and angles, you will see more than references fabricated to the term "radians" instead of "degrees". Then, what is a "radian" ? |  | The radian measure, θ, of a central bending is defined as the ratio of the length of the arc | | the angle subtends, s , divided past the radius of the circumvolve, r. | which gives arc length, s : due south = θr | 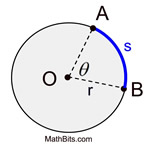
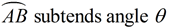
subtend = "to be opposite to" | | Ane radian is the central angle that subtends an arc length of i radius ( south = r).
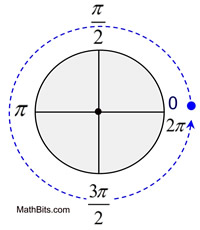
Since all circles are similar, one radian is the same value for all circles. |  Human relationship between Degrees and Radians:
In a circumvolve, the arc measure of the entire circle is 360º and the arc length of the entire circle is represented by the formula for circumference of the circle:  . . Substituting C into the formula southward = θr shows:
C = θrtwo πr = θr 2 π = θ
The arc measure of the fundamental angle of an entire circle is 360º and the radian measure of the central angle of an unabridged circle = 2π.
360º (degrees) = iiπ (radians)
|  | | 360º = 2π
(divide both sides by two)
180º = π | 360º = iiπ
(dissever both sides by 4)
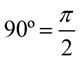 | 360º = twoπ
(divide both sides past 360)
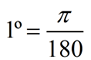
| 360º = 2π
(split both sides by 2π)
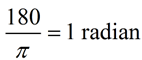
|  To modify
from degrees to radians,
multiply degrees past  | To alter
from radians to degrees,
multiply radians past 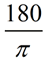 | | 1. Convert 60º to radians.

| 2. Catechumen 135º to radians.
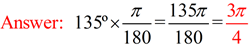 | | 3. Convert  to degrees. to degrees.
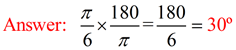
| 4. Convert  to degrees. to degrees.
 | | 5. Detect the length of an arc subtended by an angle of 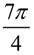 radians in a circumvolve of radius 20 centimeters. radians in a circumvolve of radius 20 centimeters.
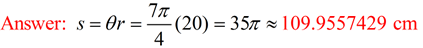
| Justify why: The length of the arc intercepted past a central angle is proportional to the radius. | The diagram at the right shows ii circles with the same center (concentric circles). Information technology has already been shown that concentric circles are similar under a dilation transformation. The ratio of similitude of the smaller circumvolve to the larger circle is:
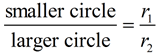 |  | | The same dilation that mapped the smaller circle onto the larger circle volition besides map the slice (sector) of the smaller circle with an arc length of s 1 onto the slice (sector) of the larger circumvolve with an arc length of s two. When the radius gets dilated by a scale factor, the arc length is besides dilated past that same scale factor. | 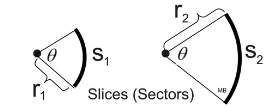 As long as the central angles are the aforementioned, the slices (sectors) will be similar. As long as the central angles are the aforementioned, the slices (sectors) will be similar. | Since corresponding parts of similar figures are in proportion, 
An equivalent proportion can be written every bit  This proportion shows that the ratio of the arc length intercepted by a central bending to the radius of the circumvolve volition ever yield the same (constant) ratio. This proportion shows that the ratio of the arc length intercepted by a central bending to the radius of the circumvolve volition ever yield the same (constant) ratio. In relation to the two arc length formulas seen on this page, both show that arc length, s, is expressed equally "some value" times the radius, r. The arc length is proportional to the radius. When θ is in degrees:  | When θ is in radians:  | Setting r = ane shows the constant of proportionality.

NOTE: The re-posting of materials (in part or whole) from this site to the Internet is copyright violation
and is not considered "off-white utilize" for educators. Delight read the "Terms of Use". |

How To Find Arc Length Of A Circle In Radians,
Source: https://mathbitsnotebook.com/Geometry/Circles/CRArcLengthRadian.html
Posted by: donaldsonskencle.blogspot.com



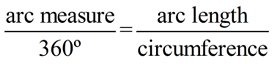
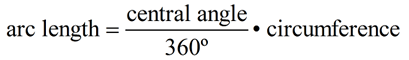



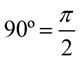
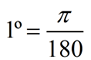
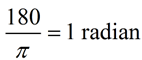


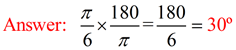

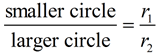

 As long as the central angles are the aforementioned, the slices (sectors) will be similar.
As long as the central angles are the aforementioned, the slices (sectors) will be similar. 
 This proportion shows that the ratio of the arc length intercepted by a central bending to the radius of the circumvolve volition ever yield the same (constant) ratio.
This proportion shows that the ratio of the arc length intercepted by a central bending to the radius of the circumvolve volition ever yield the same (constant) ratio.


0 Response to "How To Find Arc Length Of A Circle In Radians"
Post a Comment